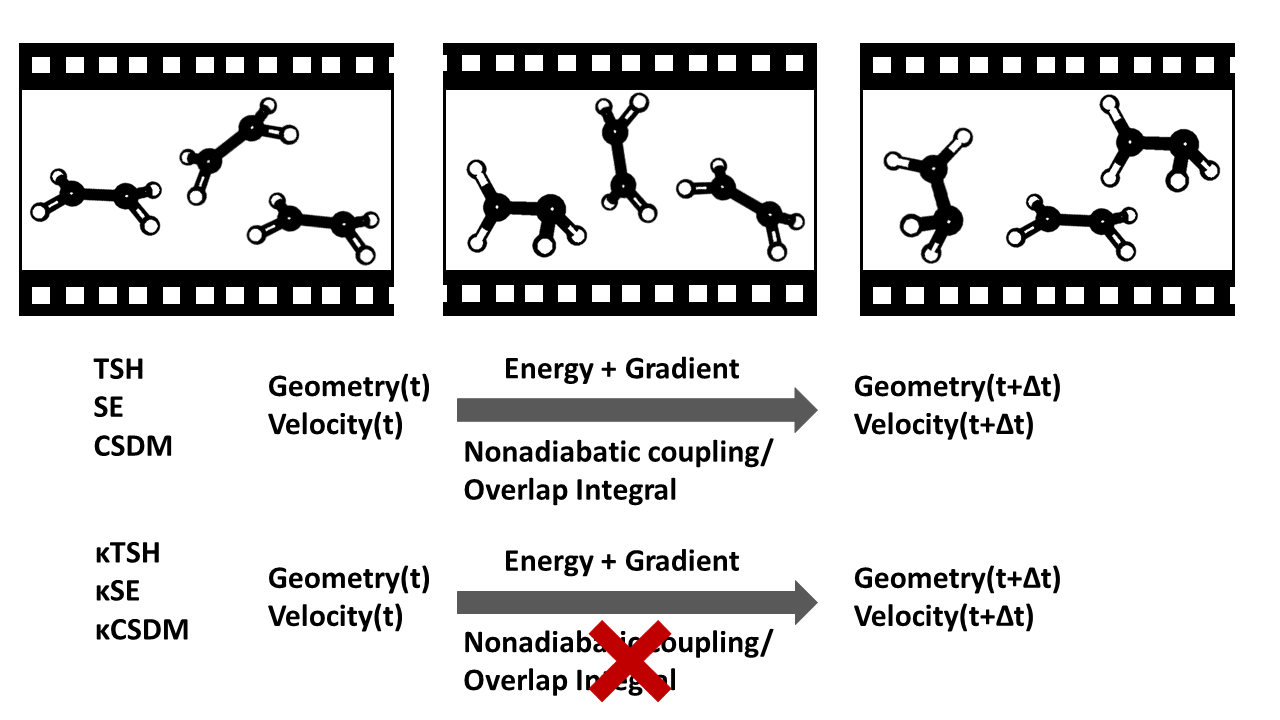
Whenever possible one prefers to perform dynamical calculations by direct dynamics, in which potential energies, their derivatives, and potential surface couplings are computed on demand by electronic structure calculations carried out side-by-side with the dynamics algorithm. For electronically nonadiabatic processes, this requires not only energies but also nonadiabatic coupling vectors (NACs), which are defined as
$$\mathbf{d}_{I J}=\left\langle\phi_I\middle|\partial / \partial \mathbf{R}\middle| \phi_J\right\rangle$$
where \(\left\langle\ldots\middle|\ldots\middle|\ldots\right\rangle\) denotes an electronic matrix element, \(\phi_I\) and \(\phi_J\) are electronic eigenfunctions, and the operator is a \(3N_\textrm{atoms}\)-dimensional gradient with respect to nuclear coordinates. The evaluation of the NACs adds to the cost, and it is often inconvenient. For example, some software will produce the electronic energies but not the NACs, and for some methods of predicting electronic energies, the NACs are unavailable because the method predicts energies without an explicit wave function corresponding to the energies. In addition to expense and inconvenience, even when NACs are available, they present problems due to origin dependence, spurious long-range couplings, and artificial translational and rotational components.
In some semiclassical multi-surface trajectory methods, like trajectory surface hopping (TSH), one only needs the component of the NAC along the current velocity direction, and in such cases one can use the time derivative of the electronic wave function along the trajectory instead of the NAC. Although, when possible, this is more efficient, it would be even more convenient to have approximations to NACs that can be evaluated straightforwardly from energies and energetic derivatives without gradients of electronic wave functions or time derivatives of electronic wave functions. In other more accurate dynamics methods, like quantum dynamics or coherent switching with decay of mixing (CSDM), one needs the whole NAC. We therefore asked, can we carry out electronically nonadiabatic dynamics using only energy information, and does this provide enough accuracy for efficient propagation of multi-surface molecular dynamics? We were able to answer this question, “YES – NACs for free!”. This work is now published:
“Nonadiabatic Dynamics Algorithms with Only Potential Energies and Gradients: Curvature-Driven Coherent Switching with Decay of Mixing and Curvature-Driven Trajectory Surface Hopping,” Y. Shu, L. Zhang, X. Chen, S. Sun, Y. Huang, and D. G. Truhlar, Journal of Chemical Theory and Computation 18, 1320-1328 (2022). doi.org/10.1021/acs.jctc.1c01080
In the above work, starting with the curvature-based approximation of Baeck and An for one-dimensional systems, we proposed a curvature-approximated time-derivative coupling formula and effective NAC applicable to polyatomic dynamics. (In independent work carried out at about the same time, do Casal et al. [doi.org/10.12688/openreseurope.13624.1] also extended the Baeck–An approximation to multiple dimensions, but in their case only for the component of the NAC along the velocity vector.) We showed how to use the time-derivative coupling formula and effective NACs to obtain curvature-driven versions of CSDM and TSH with energy-based decoherence (TSH-EDC). These curvature-driven methods are respectively called κCSDM and κTSH-EDC. In the original paper (reference above) we compared S1 population decays calculated by κCSDM to those calculated by CSDM based on NACs and CSDM based on time-derivative coupling (approximated by overlap integrals of electronic wave functions). These calculations employed SA-CASSCF electronic wave functions, and the half-lives of the three dynamical methods agree within the statistical uncertainty due to Monte Carlo sampling over initial conditions of the ensemble.
A similar comparison of SA-CASSCF S1 population decays was made for ammonia:
“Direct Nonadiabatic Dynamics of Ammonia with Curvature-Driven Coherent Switching with Decay of Mixing and with Fewest Switches with Time Uncertainty: An Illustration of Population Leaking in Trajectory Surface Hopping Due to Frustrated Hops,” X. Zhao, Y. Shu, L. Zhang, X. Xu, and D. G. Truhlar, Journal of Chemical Theory and Computation 23, 1672-1685 (2023). doi.org/10.1021/acs.jctc.2c01260
Good agreement was found between κCSDM and CSDM based on NACs.
Another check of κCSDM was carried out for photodissociation of 1,3-cyclohexadiene:
“Nonadiabatic Dynamics of 1,3-Cyclohexadiene by Curvature-Driven Coherent Switching with Decay of Mixing,” L. Zhang, Y. Shu, S. Bhaumik, X. Chen, S. Sun, Y. Huang, and D. G. Truhlar, Journal of Chemical Theory and Computation 18, 7073-7081 (2022). doi.org/10.1021/acs.jctc.2c00801
In this work we compared 42-dimensional 3-state κCSDM calculations based on XMS-CASPT2 wave functions to experiment and to earlier dynamics calculations employing NACs [Polyak et al., doi.org/10.1021/acs.jctc.9b00396], and again good agreement was obtained.
Systems with dense manifolds of electronically excited states can be challenging for electronically nonadiabatic dynamics. We compared κCSDM calculations to CSDM calculations based on NACs for total electronically nonadiabatic cross sections in high-energy 5A´collisions of ground-electronic state O2 with ground state O:
Semiclassical Multi-State Dynamics for Six Coupled 5A′ States of O + O2,” F. B. Akher, Y. Shu, Z. Varga, and D. G. Truhlar, Journal of Chemical Theory and Computation 19, 4389-4401 (2023). doi.org/10.1021/acs.jctc.3c00517
These calculations, unlike the ones already discussed, were not direct dynamics but rather were based on analytic fits to diabatic potential energy matrices based on input from 6-state XMS-CASPT2 calculations. We found very good agreement for 30 sets of initial translational and vibrational energies.
Concluding remarks
The curvature-driven methods do not require the calculations of NACs or time derivatives of the wave function. There are no spurious long-range couplings, no origin dependence, no artificial breaking of the conservation of center of mass motion and nuclear angular momentum, no phase issues, and no added expense. Electronically nonadiabatic dynamics calculations agree well with calculations based on NACs. The curvature-driven methods can be used for direct dynamics calculations of electronically nonadiabatic processes with electronic structure methods for which NACs or time derivatives are not available. They simply require adiabatic potential energy surfaces and their gradients. Both κCSDM and κTSH-EDC are available in the software packages SHARC 3.0. and SHARC-MN 2.0:
SHARC 3.0: M. Oppel, maisebastian, and schnarc. (2023). sharc-md/sharc: SHARC Release 3.0.1 (v3.0.1). Zenodo. https://doi.org/10.5281/zenodo.7896064
SHARC-MN 2.0: Y. Shu, L. Zhang, and D. G. Truhlar. (2023). SHARC-MN v2.0 (2.0). Zenodo. https://doi.org/10.5281/zenodo.7818894
